- Главная
- Разное
- Дизайн
- Бизнес и предпринимательство
- Аналитика
- Образование
- Развлечения
- Красота и здоровье
- Финансы
- Государство
- Путешествия
- Спорт
- Недвижимость
- Армия
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Неустойчивость тонких пленок, самоорганизация на поверхности презентация
Содержание
- 1. Неустойчивость тонких пленок, самоорганизация на поверхности
- 2. Нестабильность механически напряженной пленки Instability of mechanically
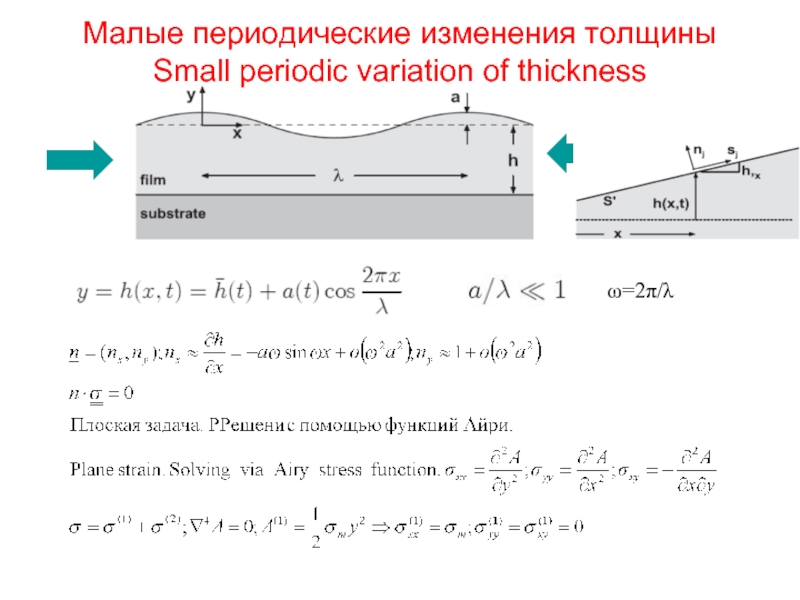
- 3. Малые периодические изменения толщины Small periodic variation of thickness ω=2π/λ
- 4. Малые периодические изменения толщины Small periodic variation of thickness
- 5. Плотность энергии и химический потенциал Energy and
- 6. Критическая длина стабильности Critical length of stability
- 7. Нестабильность напряженной пленки Asaro-Tiller--Grinfeld instability ν =
- 8. Нестабильность пленки GeSi на Si Asaro-Tiller--Grinfeld
- 9. Возмущения второго порядка Second order disturbation
- 10. Применимость приближения малых флуктуаций Applicability of small
- 11. Домашнее задание (Homework) 4 Пленка Ge толщиной
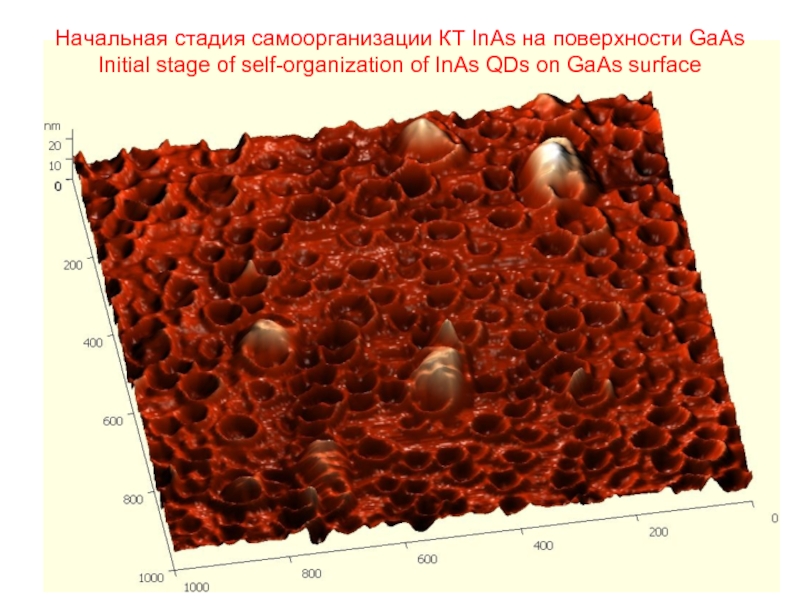
- 12. Начальная стадия самоорганизации КТ InAs на поверхности
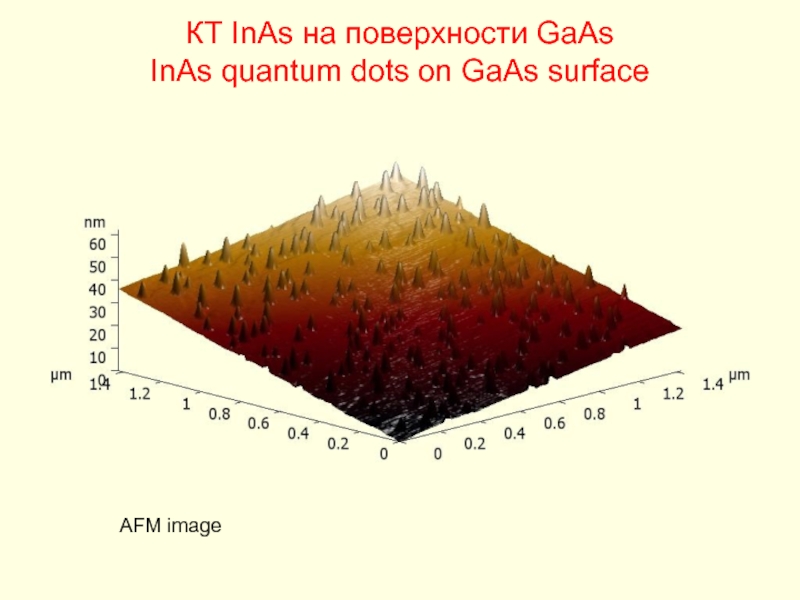
- 13. КТ InAs на поверхности GaAs InAs quantum dots on GaAs surface AFM image
- 14. Причина формирования КТ – выигрыш в упругой
- 15. Механизм Странского-Крастанова Stranski-Krastanow mechanism Размерный параметр задачи Dimensional parameter γf~1J/m2, Mf~1011Pa, εm~0.03
- 16. Механизм Странского-Крастанова Stranski-Krastanow mechanism dimensionless parameter
- 17. Соотношение вертикального и латерального размеров Optimal
- 18. Упругая энергия при изменении отношения высоты островка
- 19. Начальные стадии формирования пирамид Ge на Si
- 20. Формы островков Ge на Si Shapes of
- 21. Распределение по размерам Size distribution 0 1
- 22. Эволюция формы Shape evolution Shape transition of
- 23. Формы островков Shape evolution Typical Ge
- 24. Геометрия островков InAs на GaAs и Ge
- 25. Нуклеация пирамидальных островков Nucleation of pyramidal islands
- 26. Фасетирование островков InAs на GaAs Facetting of
- 27. Фасетирование островков Facetting of islands Зубчатая фасетированная
- 28. Энергия фасетированной поверхности Energy of facetted surface
- 29. Равновесность системы островков The energy of a
- 30. Самоорганизация QD на поверхности: Кинетика или термодинамика?
- 31. Роль условий роста Role of growth conditions
- 32. Самоорганизация квантовых точек на поверхности Self-organization of
- 33. Режимы роста гетероэпитаксиальных систем Growth modes in
- 34. Фазовая диаграмма режимов роста Phase diagram of
- 35. Домашнее задание (Homework) 5 Пленка Ge толщиной
- 36. Латеральное упорядочение островков Энергия латерального взаимодействия островков
- 37. Энергия латерального взаимодействия островков на поверхности Normalized
- 38. Латеральное упорядочение островков InAs на GaAs Ordering
- 39. Искусственное упорядочение квантовых точек AFM images of
Слайд 1Наномеханика
Nanomechanics of materials and systems
Lecture 5
Неустойчивость тонких пленок, само-организация на
Surface instability and self-organization
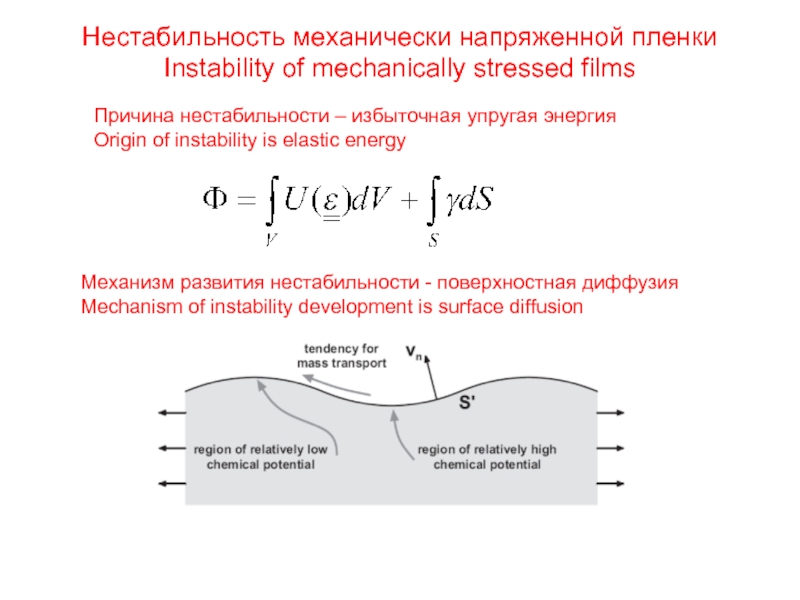
Слайд 2Нестабильность механически напряженной пленки
Instability of mechanically stressed films
Механизм развития нестабильности -
Mechanism of instability development is surface diffusion
Причина нестабильности – избыточная упругая энергия
Origin of instability is elastic energy
Слайд 5Плотность энергии и химический потенциал
Energy and chemical potential
Увеличение площади поверхности квадратично
Химический потенциал (Chemical potential)
Слайд 6Критическая длина стабильности
Critical length of stability
Пусть a зависит от времени
Изменение свободной
Free energy over a period
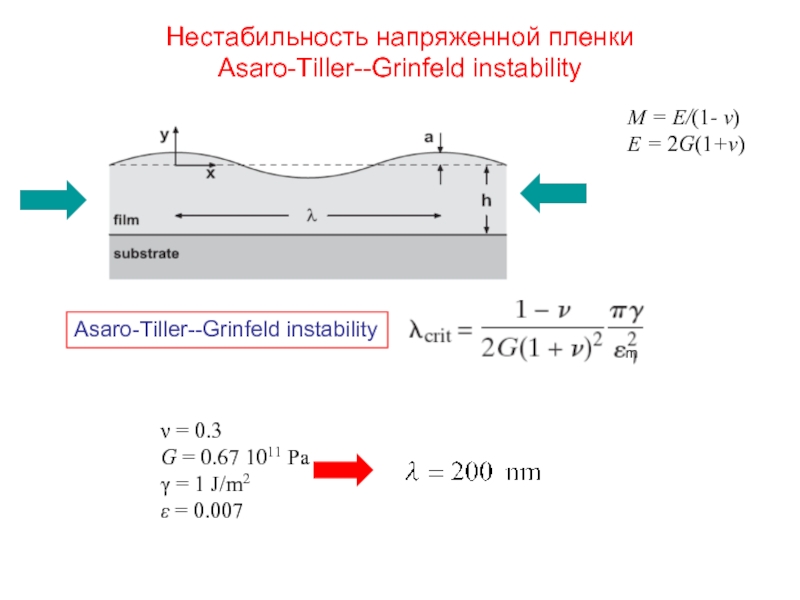
Нестабильность Азаро-Тиллера—Гринфельда
Asaro-Tiller-Grinfeld instability
Слайд 7Нестабильность напряженной пленки
Asaro-Tiller--Grinfeld instability
ν = 0.3
G = 0.67 1011 Pa
γ =
ε = 0.007
Asaro-Tiller--Grinfeld instability
M = E/(1- ν)
E = 2G(1+ν)
Слайд 8Нестабильность пленки GeSi на Si
Asaro-Tiller--Grinfeld instability of GeSi on Si
Transmission
a
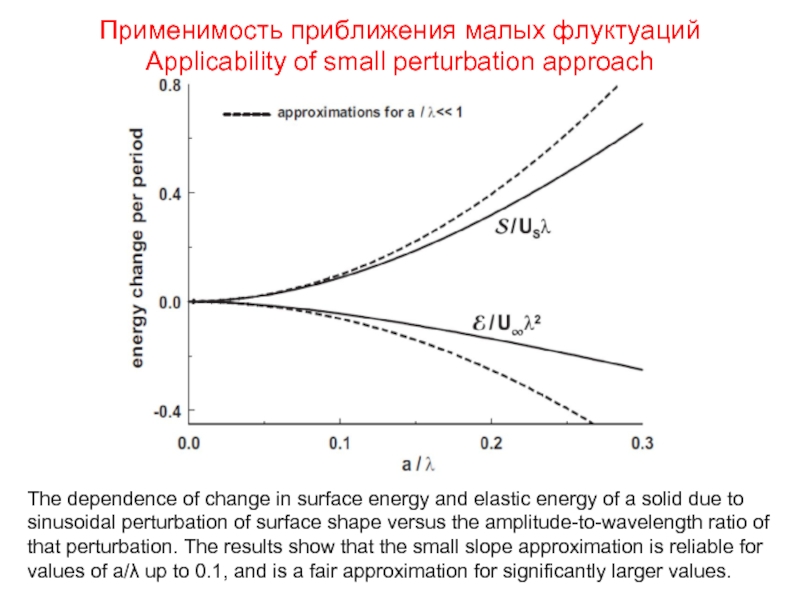
Слайд 10Применимость приближения малых флуктуаций
Applicability of small perturbation approach
The dependence of change
Слайд 11Домашнее задание (Homework) 4
Пленка Ge толщиной 4 нм выращена эпитаксиально на
Определить (determine)
1. Анизотропию кривизны структуры по Стони Anisotropy of Stoney curvature (if any?)
2. Критическую длину Asaro-Tiller-Grinfeld нестабильности пленки (γ = 1 J/m2). Asaro-Tiller-Grinfeld critical length (γ = 1 J/m2).
Слайд 12Начальная стадия самоорганизации КТ InAs на поверхности GaAs Initial stage of
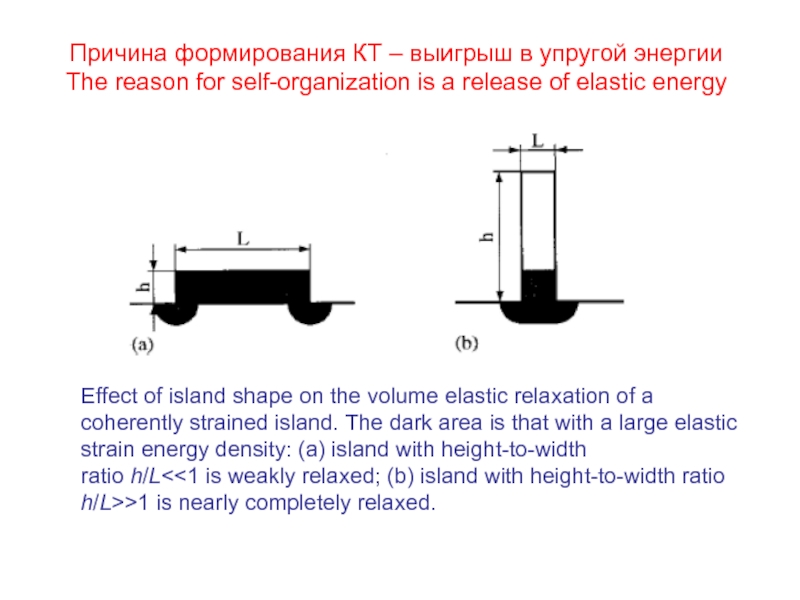
Слайд 14Причина формирования КТ – выигрыш в упругой энергии The reason for
Effect of island shape on the volume elastic relaxation of a coherently strained island. The dark area is that with a large elastic strain energy density: (a) island with height-to-width
ratio h/L<<1 is weakly relaxed; (b) island with height-to-width ratio h/L>>1 is nearly completely relaxed.
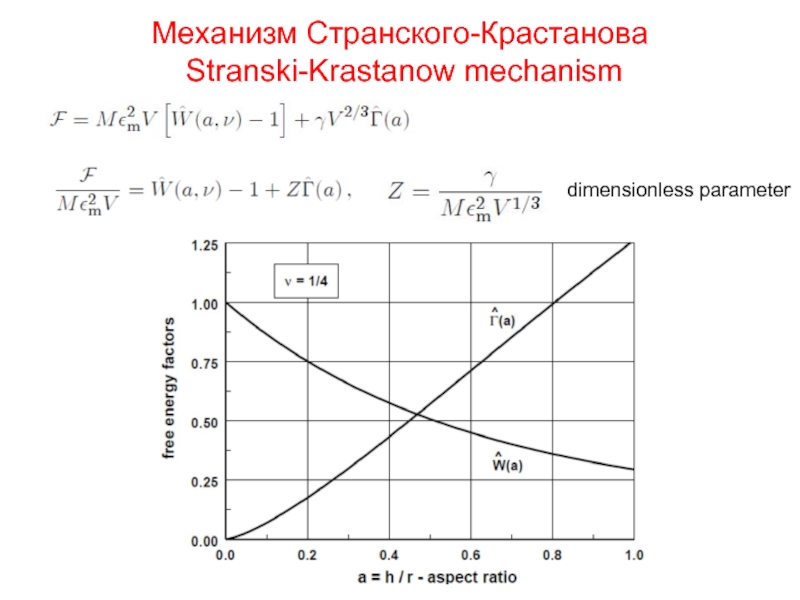
Слайд 15Механизм Странского-Крастанова
Stranski-Krastanow mechanism
Размерный параметр задачи
Dimensional parameter
γf~1J/m2, Mf~1011Pa, εm~0.03
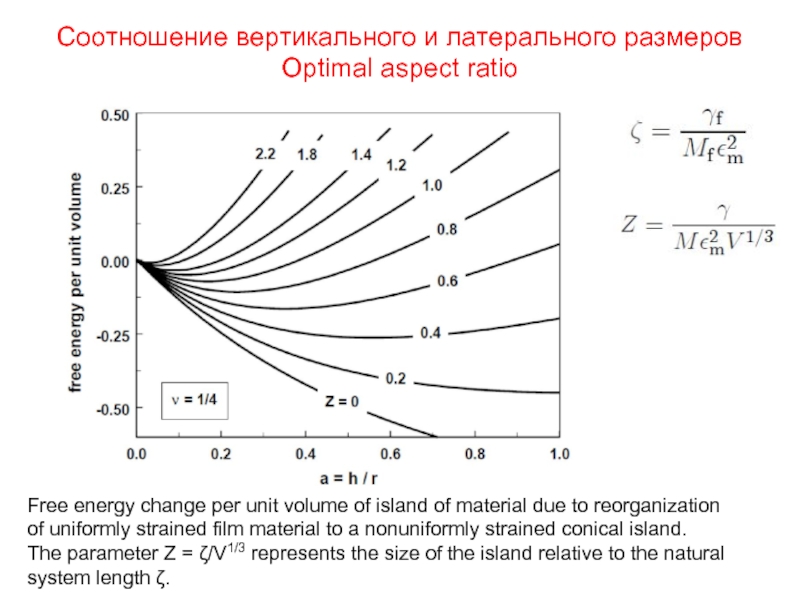
Слайд 17Соотношение вертикального и латерального размеров
Optimal aspect ratio
Free energy change per
of uniformly strained film material to a nonuniformly strained conical island.
The parameter Z = ζ/V1/3 represents the size of the island relative to the natural system length ζ.
Слайд 18Упругая энергия при изменении отношения высоты островка к его основанию Elastic energy
Total, normalized energy as a function of island aspect ratio (one with a log scale on the
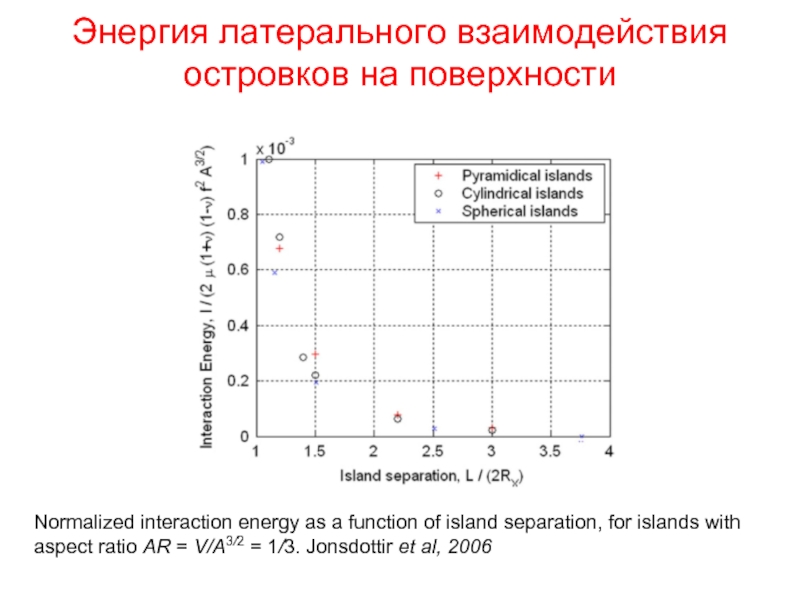
abscissa is shown to convey more detail in the transition regime). Data points represent a mix of spherical caps and pucks; all fall along the same curve. For aspect ratios larger than approximately 0.25, the energy (when scaled as such) becomes independent of aspect ratio. Jonsdottir et al, 2006
Слайд 19Начальные стадии формирования пирамид Ge на Si Initial stages of formation of
STM images of the surface evolution during growth of a Ge layer on Si (001). In panels (a)–(d), the Ge coverage grows from 2.8 to 4.0 ML’s. During growth (a)–(c) first mounds
(prepyramids) develop, then these convert (d) to {105} facetted pyramids. From Vailionis et al., 2000.
Слайд 20Формы островков Ge на Si
Shapes of Ge islands on Si
The size
from Vailionis et al., 2000.
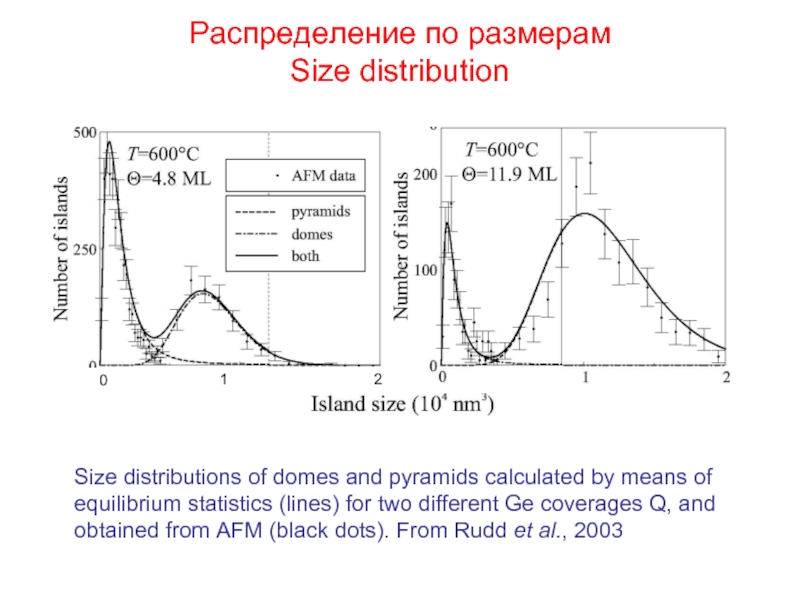
Слайд 21Распределение по размерам
Size distribution
0
1
2
Size distributions of domes and pyramids calculated by
Слайд 22Эволюция формы
Shape evolution
Shape transition of Ge or SiGe islands grown on
Слайд 23Формы островков
Shape evolution
Typical Ge island shapes obtained by STM during
Слайд 24Геометрия островков InAs на GaAs и Ge на Si Geometry of InAs
Geometry of an InAs pyramid over an InAs wetting layer deposited on a GaAs(001) surface.
3D STM image (a) and schematic top and side views (b) of a 3D island formed during the deposition of pure Ge on Si(001). All faces of the island are {105} facets. The height of the island is 2.8 nm while the base dimensions are of the order of 20 and 40 nm. Due to frequently observed elongation of the islands—that is either in [100] or [010] direction—the island’s shape resembles that of a “hut”. The vertical scale of the STM image is exaggerated: the islands actually are fairly Kat with an 11.3° tilt angle of the facets as it is shown in (b) (after Mo et al. and Teichert et al.).
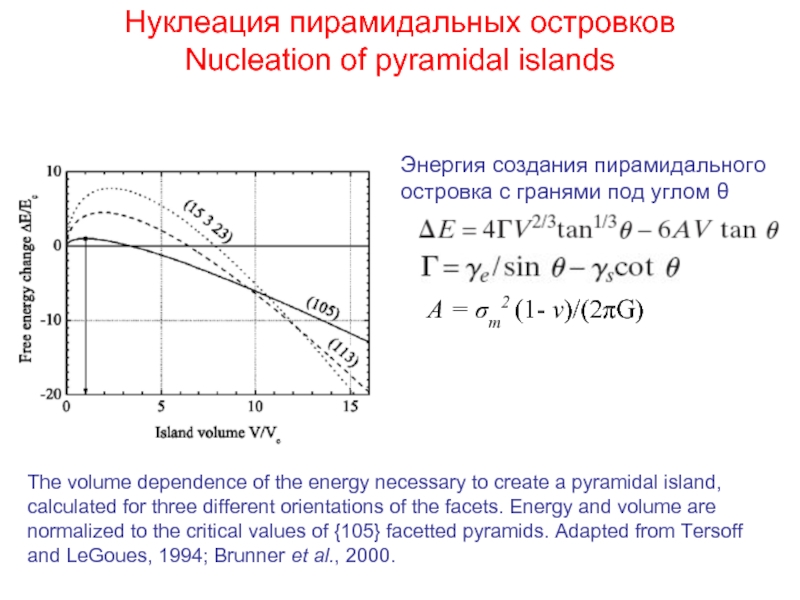
Слайд 25Нуклеация пирамидальных островков
Nucleation of pyramidal islands
The volume dependence of the energy
Энергия создания пирамидального островка с гранями под углом θ
A = σm2 (1- ν)/(2πG)
Слайд 26Фасетирование островков InAs на GaAs
Facetting of InAs islands on GaAs
Facetting of
Слайд 27Фасетирование островков
Facetting of islands
Зубчатая фасетированная поверхность. Эффективные силы противоположного знака приложены
Полная энергия (Total energy)
The balance of forces acting on a crystal edge. Forces caused by the intrinsic surface stress τ are balanced by the force G acting from the bulk of the crystal. According to Newton’s third law, the reaction F=-G is acting from the surface layer on the bulk of the crystal, resulting in the strain field. The inset in the middle depicts the force balance, which is similar for a liquid and for a crystal.
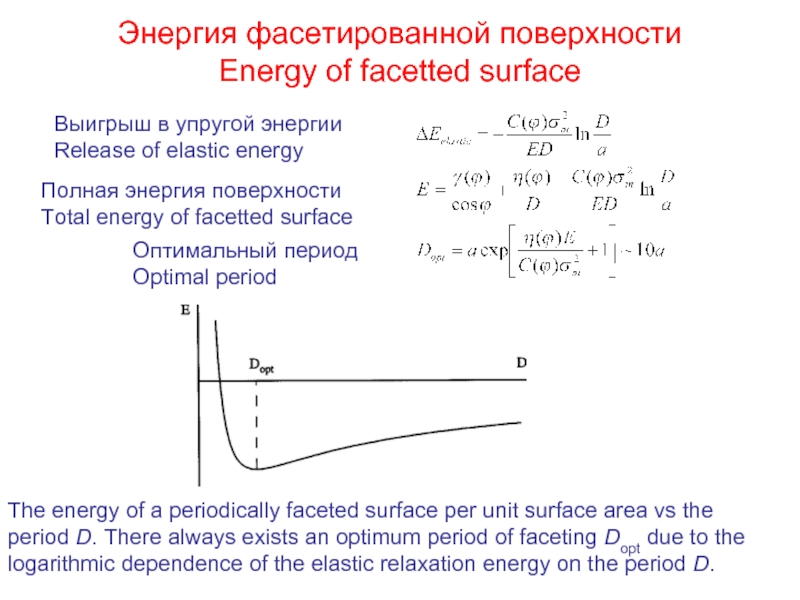
Слайд 28Энергия фасетированной поверхности
Energy of facetted surface
The energy of a periodically faceted
Выигрыш в упругой энергии
Release of elastic energy
Полная энергия поверхности
Total energy of facetted surface
Оптимальный период Optimal period
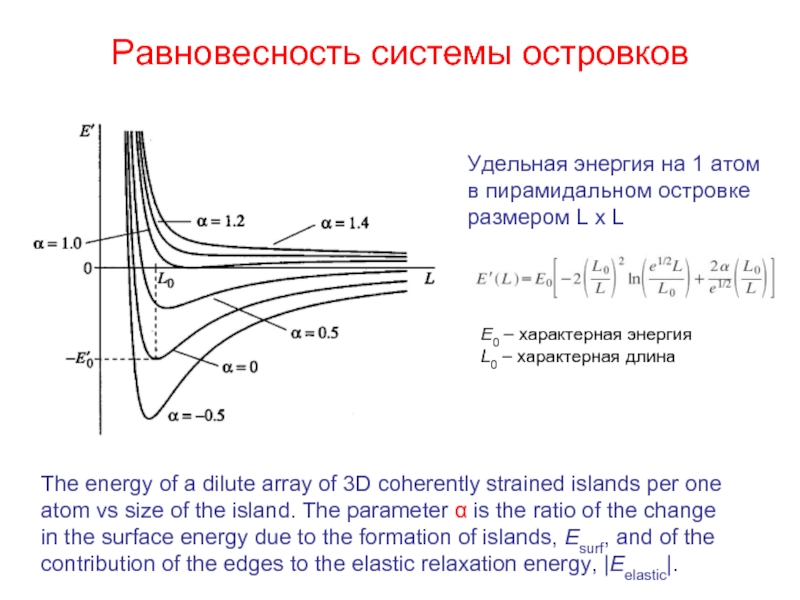
Слайд 29Равновесность системы островков
The energy of a dilute array of 3D coherently
Удельная энергия на 1 атом в пирамидальном островке размером L x L
E0 – характерная энергия
L0 – характерная длина
Слайд 30Самоорганизация QD на поверхности:
Кинетика или термодинамика?
Self-organization: kinetics or thermodynamics?
Формирование квантовых точек
Термодинамические модели в целом описывают наблюдаемые явления Thermodynamics describes the process reasonably well
Роль кинетики зависит от конкретной системы и условий формирования квантовых точек Role of kinetics is specific for systems and conditions
Для большого числа практически важных полупроводниковых гетеросистем разработаны рецепты воспроизводимого получения массивов квантовых точек на поверхности Technology has been developed for reliable production of quantum dots in different systems
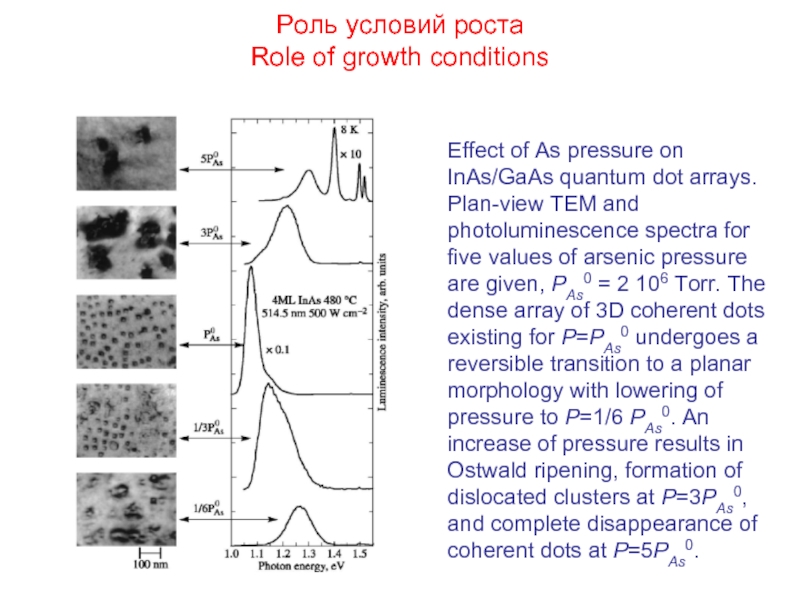
Слайд 31Роль условий роста
Role of growth conditions
Effect of As pressure on InAs/GaAs
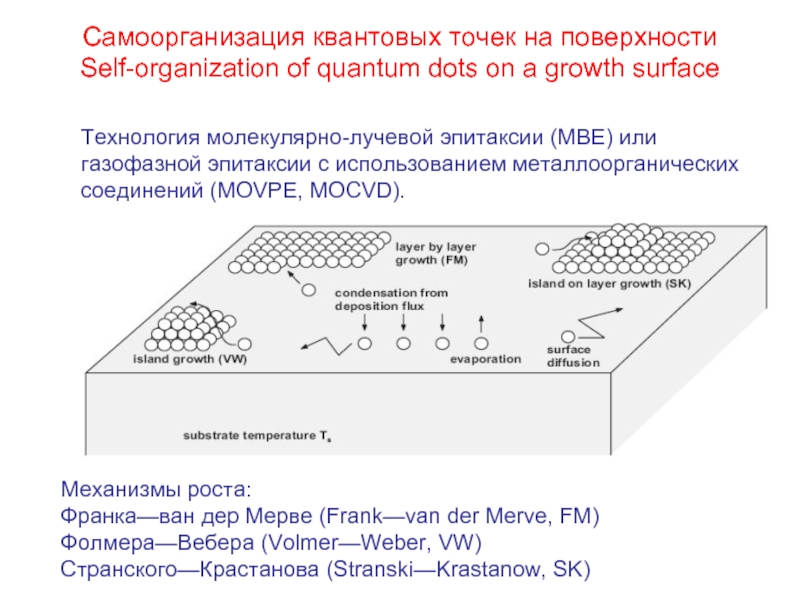
Слайд 32Самоорганизация квантовых точек на поверхности Self-organization of quantum dots on a growth
Технология молекулярно-лучевой эпитаксии (MBE) или газофазной эпитаксии с использованием металлоорганических соединений (MOVPE, MOCVD).
Механизмы роста:
Франка—ван дер Мерве (Frank—van der Merve, FM)
Фолмера—Вебера (Volmer—Weber, VW)
Странского—Крастанова (Stranski—Krastanow, SK)
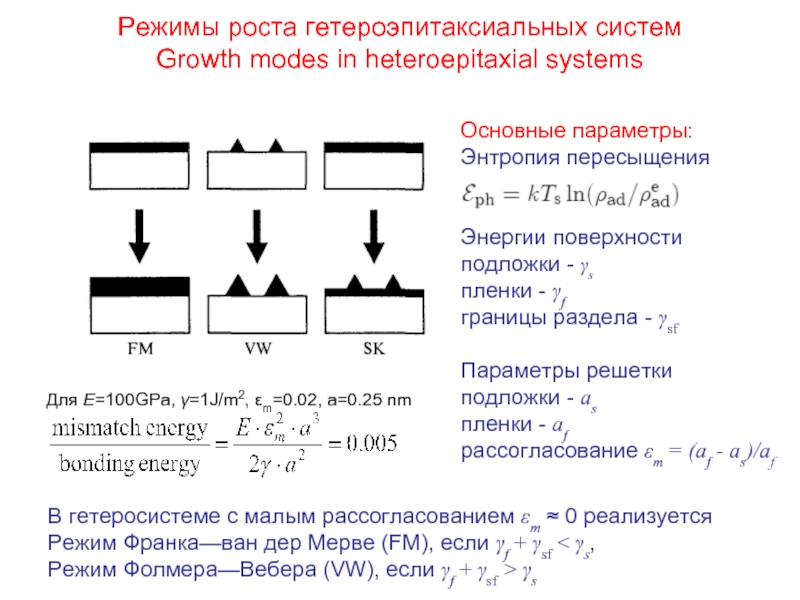
Слайд 33Режимы роста гетероэпитаксиальных систем
Growth modes in heteroepitaxial systems
Основные параметры:
Энтропия пересыщения
Энергии поверхности
подложки
пленки - γf
границы раздела - γsf
Параметры решетки
подложки - as
пленки - af
рассогласование εm = (af - as)/af
В гетеросистеме с малым рассогласованием εm ≈ 0 реализуется
Режим Франка—ван дер Мерве (FM), если γf + γsf < γs,
Режим Фолмера—Вебера (VW), если γf + γsf > γs
Для Е=100GPa, γ=1J/m2, εm=0.02, a=0.25 nm
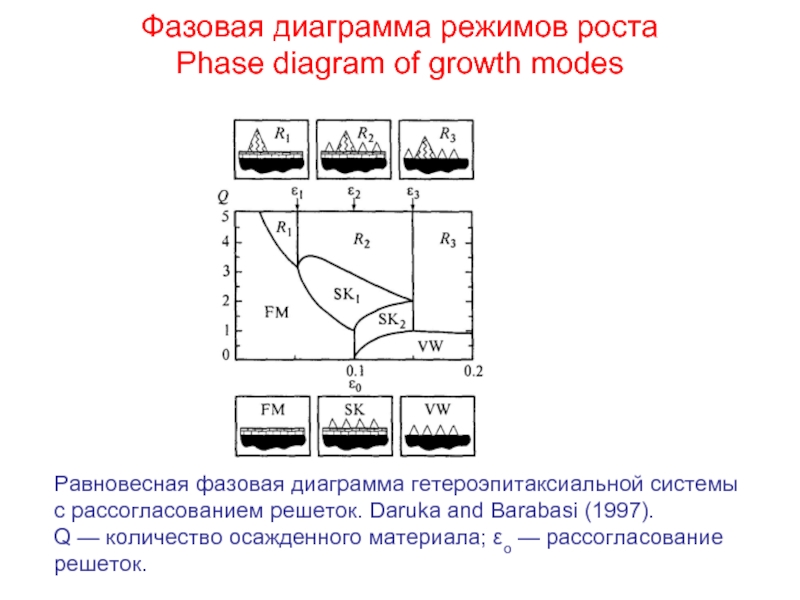
Слайд 34Фазовая диаграмма режимов роста
Phase diagram of growth modes
Равновесная фазовая диаграмма гетероэпитаксиальной
Q — количество осажденного материала; εо — рассогласование решеток.
Слайд 35Домашнее задание (Homework) 5
Пленка Ge толщиной 4 нм выращена эпитаксиально на
Определить (determine)
4.2. Критическую длину Asaro-Tiller-Grinfeld нестабильности пленки (γ = 1 J/m2). Asaro-Tiller-Grinfeld critical length (γ = 1 J/m2).
5. Характерный размер квантовых точек Ge Characteristic size of Ge quantum dots
Слайд 36Латеральное упорядочение островков
Энергия латерального взаимодействия островков
R – расстояние между островками;
V –
(mx ; my) – единичный вектор на поверхности;
B, C – константы;
для изотропной среды В2 = 0, изотропное дипольное отталкивание;
для анизотропной среды возможно притяжение по “мягким” направлениям, обычно <100>.
Слайд 37Энергия латерального взаимодействия островков на поверхности
Normalized interaction energy as a function
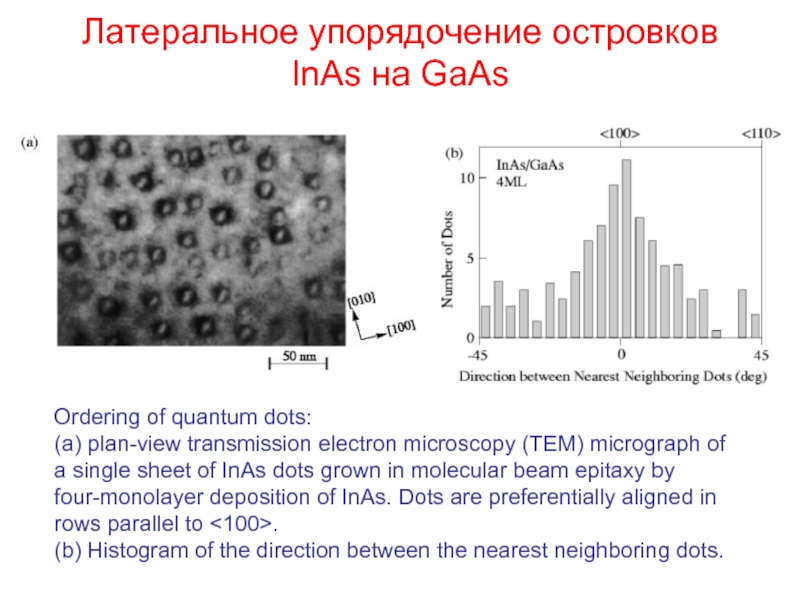
Слайд 38Латеральное упорядочение островков InAs на GaAs
Ordering of quantum dots:
(a) plan-view
Слайд 39Искусственное упорядочение квантовых точек
AFM images of the surface of a Ge
Проблемой самоорганизации КТ является статистический разброс размеров
Латеральное самоупорядочение КТ на поверхности малоэффективно