- Главная
- Разное
- Дизайн
- Бизнес и предпринимательство
- Аналитика
- Образование
- Развлечения
- Красота и здоровье
- Финансы
- Государство
- Путешествия
- Спорт
- Недвижимость
- Армия
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
The Chain Rule презентация
Содержание
- 1. The Chain Rule
- 2. Barnett/Ziegler/Byleen Business Calculus 11e Composite Functions Definition:
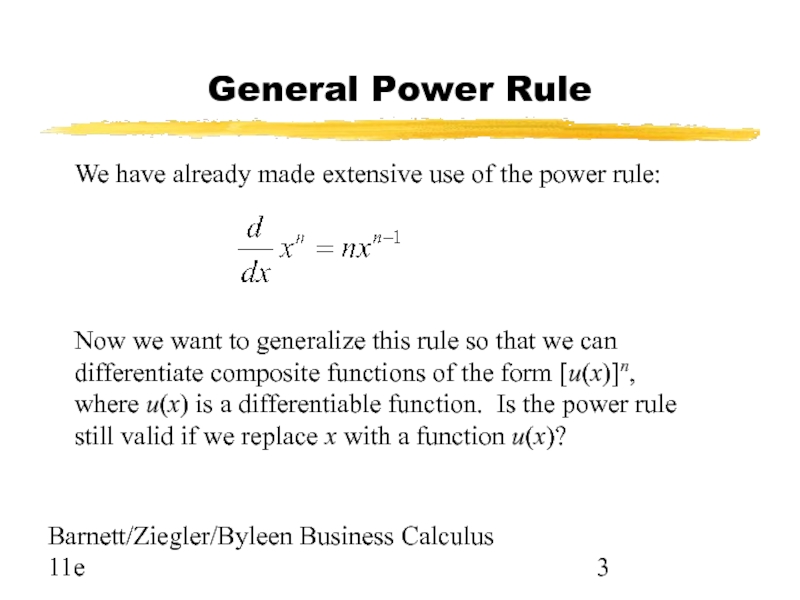
- 3. Barnett/Ziegler/Byleen Business Calculus 11e General Power Rule
- 4. Barnett/Ziegler/Byleen Business Calculus 11e Example Let u(x)
- 5. Barnett/Ziegler/Byleen Business Calculus 11e Example Let u(x)
- 6. Barnett/Ziegler/Byleen Business Calculus 11e Generalized Power Rule
- 7. Barnett/Ziegler/Byleen Business Calculus 11e Chain Rule Chain
- 8. Barnett/Ziegler/Byleen Business Calculus 11e Generalized Derivative Rules
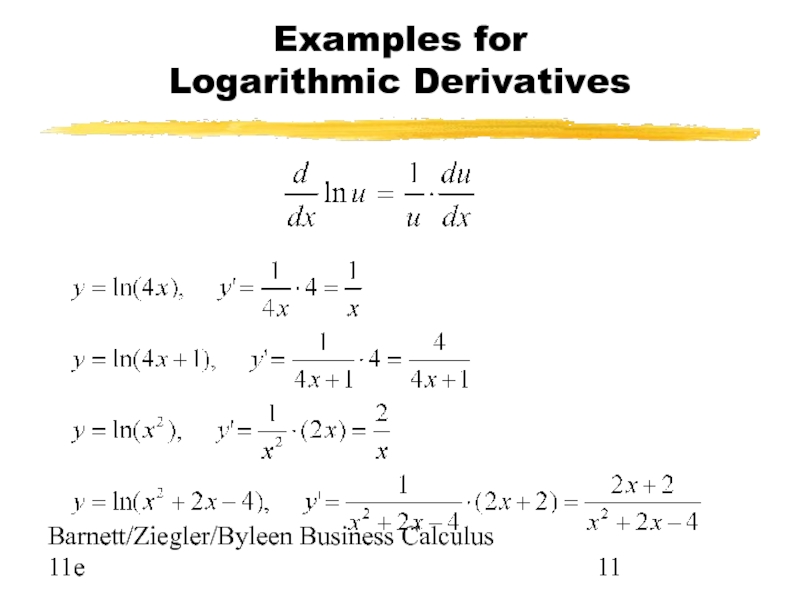
- 9. Barnett/Ziegler/Byleen Business Calculus 11e Examples for the
- 10. Barnett/Ziegler/Byleen Business Calculus 11e Examples for Exponential Derivatives
- 11. Barnett/Ziegler/Byleen Business Calculus 11e Examples for Logarithmic Derivatives
Слайд 1Barnett/Ziegler/Byleen Business Calculus 11e
Learning Objectives for Section 11.4
The Chain Rule
The
The student will be able to apply the general power rule.
The student will be able to apply the chain rule.
Слайд 2Barnett/Ziegler/Byleen Business Calculus 11e
Composite Functions
Definition: A function m is a composite
m(x) = f [g(x)]
The domain of m is the set of all numbers x such that x is in the domain of g and g(x) is in the domain of f.
Слайд 3Barnett/Ziegler/Byleen Business Calculus 11e
General Power Rule
We have already made extensive use
Now we want to generalize this rule so that we can differentiate composite functions of the form [u(x)]n, where u(x) is a differentiable function. Is the power rule still valid if we replace x with a function u(x)?
Слайд 4Barnett/Ziegler/Byleen Business Calculus 11e
Example
Let u(x) = 2x2 and f (x) =
(a) 3[u(x)]2 (b) 3[u’(x)]2 (c) 3[u(x)]2 u’(x)
Слайд 5Barnett/Ziegler/Byleen Business Calculus 11e
Example
Let u(x) = 2x2 and f (x) =
(a) 3[u(x)]2 (b) 3[u’(x)]2 (c) 3[u(x)]2 u’(x)
We know that f ’(x) = 48x5.
(a) 3[u(x)]2 = 3(2x2)2 = 3(4x4) = 12 x4. This is not correct.
(b) 3[u’(x)]2 = 3(4x)2 = 3(16x2) = 48x2. This is not correct.
(c) 3[u(x)]2 u’(x) = 3[2x2]2(4x) = 3(4x4)(4x) = 48x5. This is the correct choice.
Слайд 6Barnett/Ziegler/Byleen Business Calculus 11e
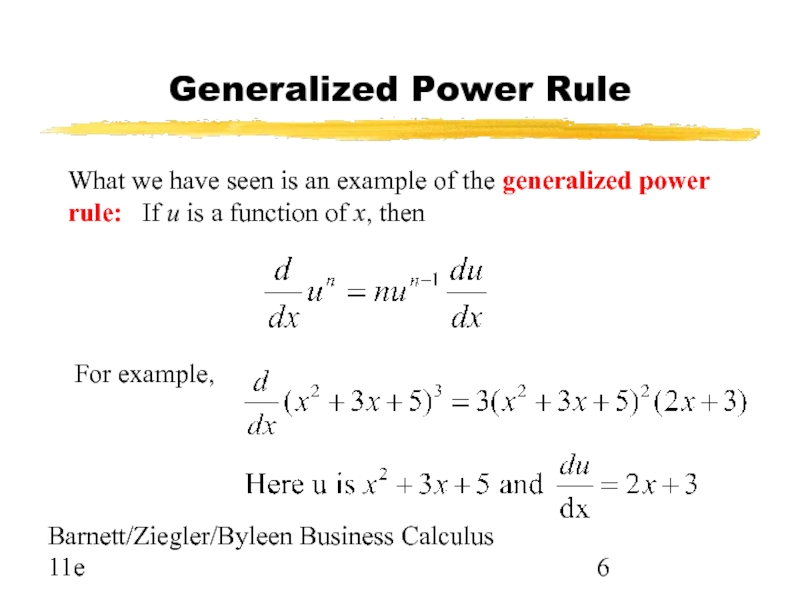
Generalized Power Rule
What we have seen is an
For example,
Слайд 7Barnett/Ziegler/Byleen Business Calculus 11e
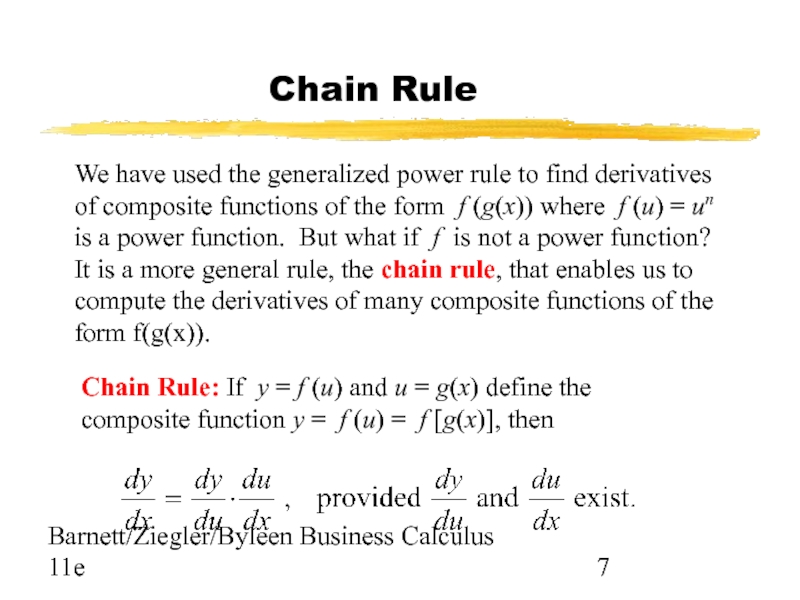
Chain Rule
Chain Rule: If y = f (u)
We have used the generalized power rule to find derivatives of composite functions of the form f (g(x)) where f (u) = un is a power function. But what if f is not a power function? It is a more general rule, the chain rule, that enables us to compute the derivatives of many composite functions of the form f(g(x)).
Слайд 8Barnett/Ziegler/Byleen Business Calculus 11e
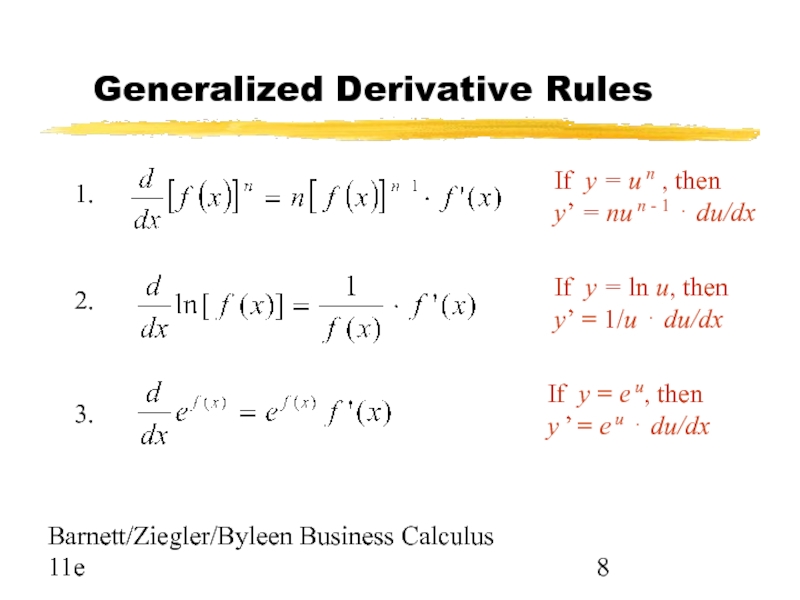
Generalized Derivative Rules
1.
2.
3.
If y = u n ,
If y = ln u, then
y’ = 1/u ⋅ du/dx
If y = e u, then
y ’ = e u ⋅ du/dx
Слайд 9Barnett/Ziegler/Byleen Business Calculus 11e
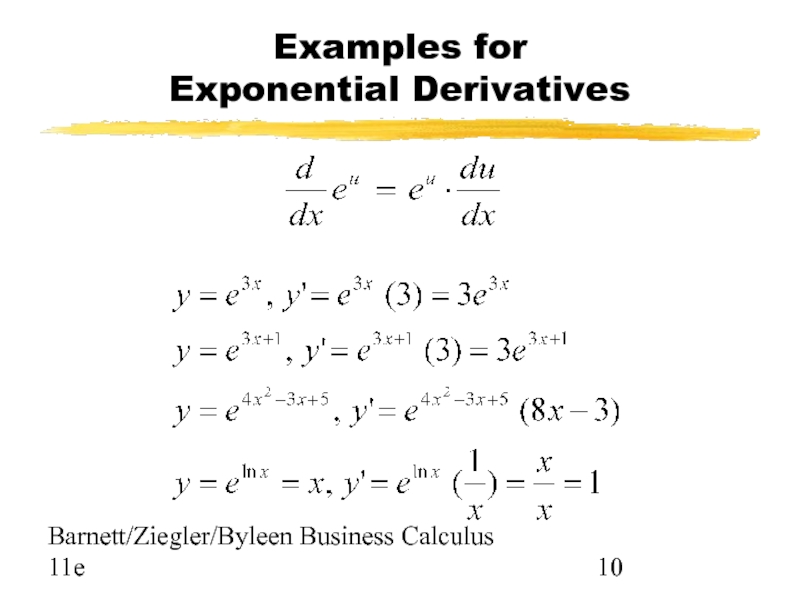
Examples for the Power Rule
Chain rule terms are

![Barnett/Ziegler/Byleen Business Calculus 11eExampleLet u(x) = 2x2 and f (x) = [u(x)]3 = 8x6. Which](/img/tmb/5/421005/6f6d723b7c9fc559e30683444a188218-800x.jpg)
![Barnett/Ziegler/Byleen Business Calculus 11eExampleLet u(x) = 2x2 and f (x) = [u(x)]3 = 8x6. Which](/img/tmb/5/421005/f92aa40fc9150bccef2b60d6c22672db-800x.jpg)