- Главная
- Разное
- Дизайн
- Бизнес и предпринимательство
- Аналитика
- Образование
- Развлечения
- Красота и здоровье
- Финансы
- Государство
- Путешествия
- Спорт
- Недвижимость
- Армия
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Mathematical Induction презентация
Содержание
- 1. Mathematical Induction
- 2. Question 0. A continuous function f is
- 3. Question 0+. A function f is defined
- 4. Mathematical Induction Let Sn, n = 1,2,3,…
- 5. Question 1. Using the Principle of Mathematical
- 6. Now, the principle of mathematical induction tells
- 7. Answers to Questions from Light #3:
- 8. Calculus++ Also known as Hysterical Calculus
- 9. Question 1b. Using the Principle of Mathematical
- 10. Now, the principle of mathematical induction tells
- 11. Question 3a. Calculate the following sum Solution. We have
- 12. Question 5. Using the formula for the
- 13. Similar calculations yield the following formula for the derivative of arccot x. Hence
- 14. Question 6. Use the Cauchy criterion to
- 15. Thus we set Therefore Thus, the sequence
- 16. Picture of the Week All ICEF students are of the same height
- 17. Question 4. Let f (x) be a
- 18. b) Is it possible that there exists
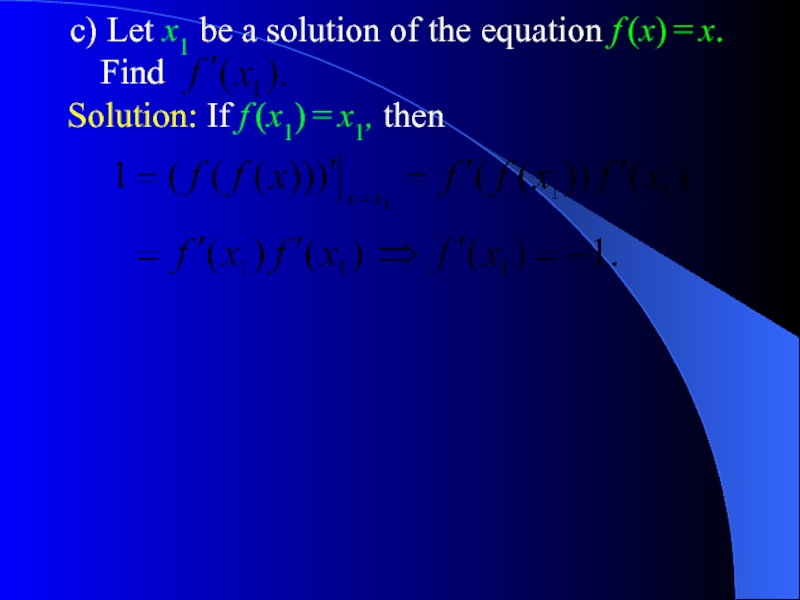
- 19. c) Let x1 be a solution of
- 20. Answers to Questions from Seminar 3. Questions
Слайд 2Question 0. A continuous function f is defined on the interval
How many functions like that exist?
Solution. The function f (x) = 0 only if x = 0.
At all other points either f (x) = x or f (x) = – x.
Since the function f is continuous, it obtains values of the same sign on each of the intervals [−1,0) and (0,1].
Therefore, there are exactly four possible cases:
Слайд 3Question 0+. A function f is defined on the interval [−1,1],
How many functions like that exist if it is known that x = ½ is the only point where f is not continuous?
Слайд 4Mathematical Induction
Let Sn, n = 1,2,3,… be statements involving positive integer
Suppose that
1. S1 is true.
2. If Sk is true, then Sk +1 is also true.
Then Sn is true for all positive integer numbers n.
Слайд 5Question 1. Using the Principle of Mathematical Induction show that
Solution. Step
Step 2. Let as assume that the formula is
Our aim is to show, that in this case the formula is also correct for n = k + 1.
case n = 1, because
correct for n = k:
for any n = 1,2,3,….
Слайд 6Now, the principle of mathematical induction tells us that our formula
We have
Слайд 7 Answers to Questions from Light #3:
Functions and Limits
Question 2:
Question 4:
Question
Question 6:
Question 5:
e) the open first quadrant
e) I and III
b) a nonconstant function
is positive for all x > 0
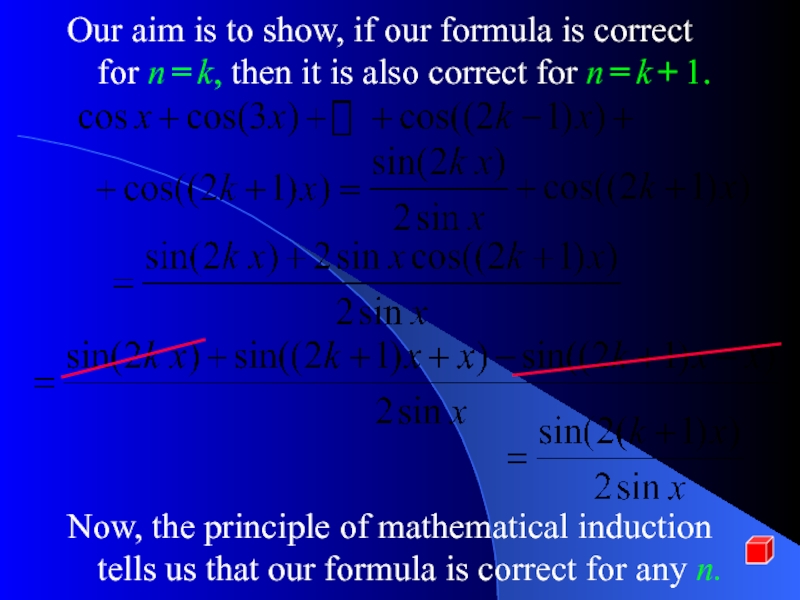
Слайд 9Question 1b. Using the Principle of Mathematical Induction show that
Solution. Step
case n = 1, because
for any n = 1,2,3,….
And hence
Step 2. Let as assume that the formula is
correct for n = k:
Слайд 10Now, the principle of mathematical induction tells us that our formula
Our aim is to show, if our formula is correct for n = k, then it is also correct for n = k + 1.
Слайд 12Question 5. Using the formula for the derivative of inverse function
Solution. Using the formula
we obtain the following formula for the derivative of arcsin x:
The range of arcsin x is the interval
Therefore cos(arcsin x) is always non-negative.
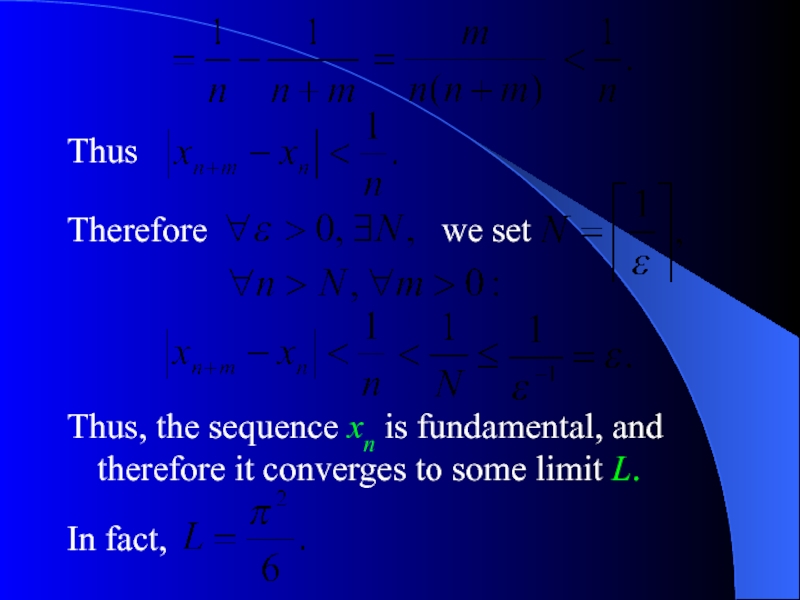
Слайд 14Question 6. Use the Cauchy criterion to show
converges.
Solution: It is sufficient
We have
that the sequence
Слайд 15Thus
we set
Therefore
Thus, the sequence xn is fundamental, and therefore it converges
In fact,
Слайд 17Question 4. Let f (x) be a differentiable function such that
a) Is it possible that there exists a number a such that
Solution: Differentiate the equation f (f (x)) = x to obtain
Therefore, there are no points a such that
because at a point like that
Слайд 18b) Is it possible that there exists a number a such
Solution: The Mean Value Theorem tells us that
Therefore, there are no points a such that
for some point c: 0 < c < 1.
otherwise there would be a point b somewhere between c and a such that

![Question 0. A continuous function f is defined on the interval [−1,1], and f 2(x)](/img/tmb/1/28112/b39284e6f5b0f1bd3dd0730c69f652d2-800x.jpg)
![Question 0+. A function f is defined on the interval [−1,1], and f 2(x) =](/img/tmb/1/28112/6d630d61f44cf5ac6644016fa1f78389-800x.jpg)