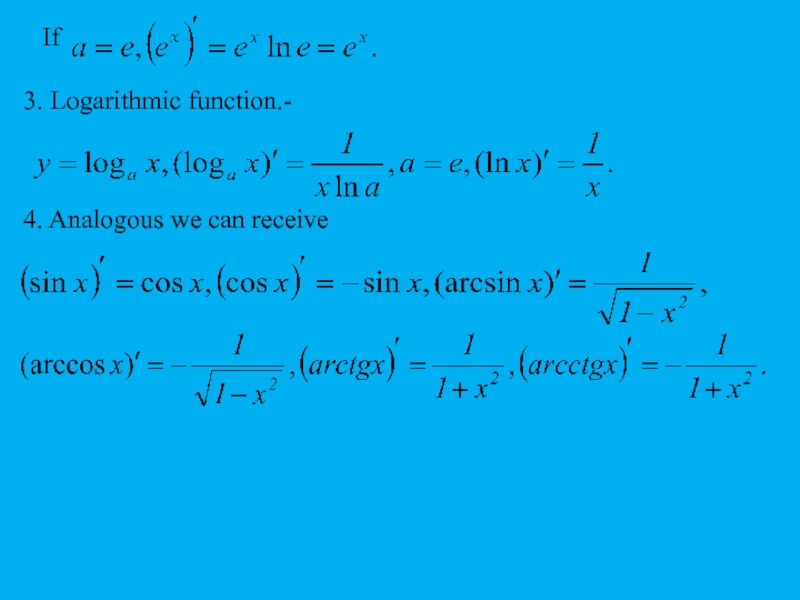- Главная
- Разное
- Дизайн
- Бизнес и предпринимательство
- Аналитика
- Образование
- Развлечения
- Красота и здоровье
- Финансы
- Государство
- Путешествия
- Спорт
- Недвижимость
- Армия
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Differential calculus of the function of one variable презентация
Содержание
- 1. Differential calculus of the function of one variable
- 2. The derivative of the function
- 3. If we designate
- 4. So we can say: the geometrical meaning
- 5. From this system taking into account that
- 6. Note, that if the following condition takes
- 7. The difference form of the continuity condition.
- 8. Definition.-
- 9. The proof of sufficiency.- Let it is
- 10. Note, that the quantities
- 11. Geometric meaning of the differential of the
- 12. The rules of calculation of derivatives of
- 13. If 3. Logarithmic function.- 4. Analogous we can receive
Слайд 1DIFFERENTIAL CALCULUS OF THE FUNCTION OF ONE VARIABLE
Eduard А.
Professor of the Moscow State University of Economics, Statistics and Informatics
E-mail: EGevorkyan@mesi.ru, gevor_mesi@mail.ru
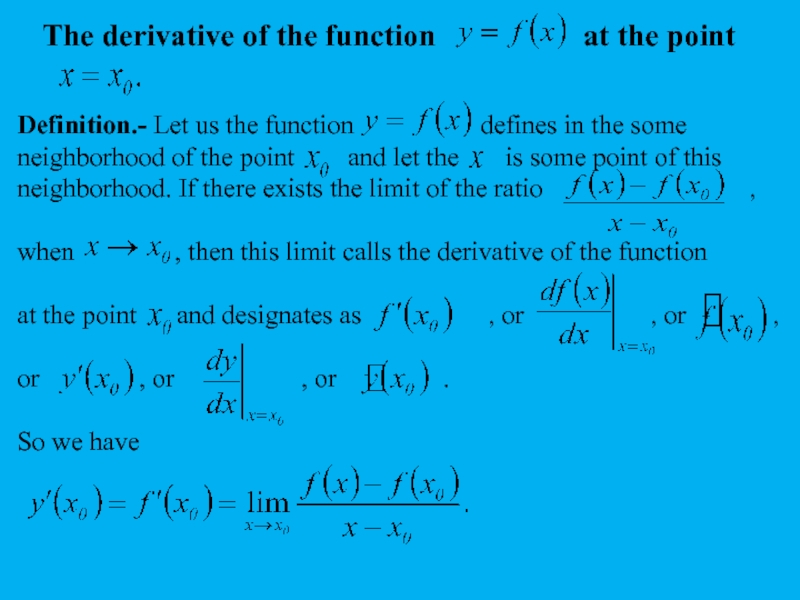
Слайд 2The derivative of the function
Definition.- Let us the function defines in the some
neighborhood of the point and let the is some point of this
neighborhood. If there exists the limit of the ratio ,
when , then this limit calls the derivative of the function
at the point and designates as , or , or ,
or , or , or .
So we have
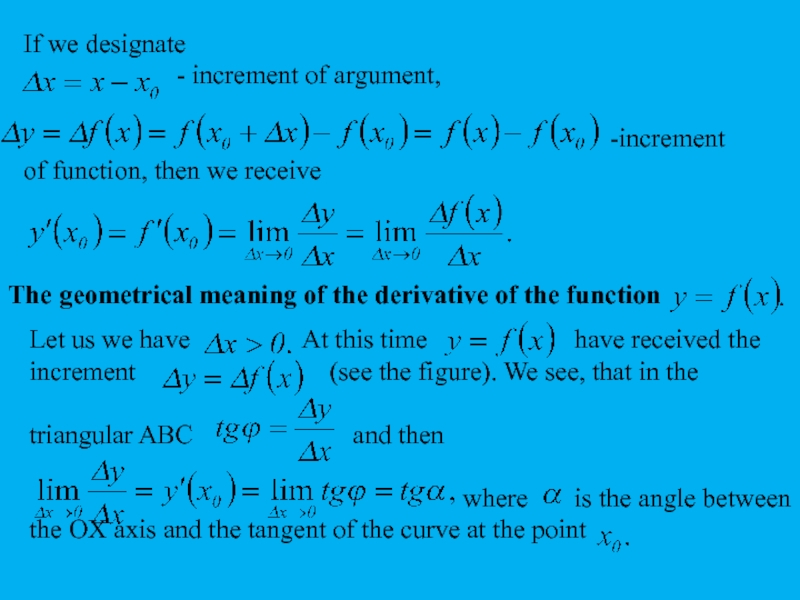
Слайд 3If we designate
-increment
of function, then we receive
The geometrical meaning of the derivative of the function
Let us we have At this time have received the
increment (see the figure). We see, that in the
triangular ABC and then
where is the angle between
the OX axis and the tangent of the curve at the point
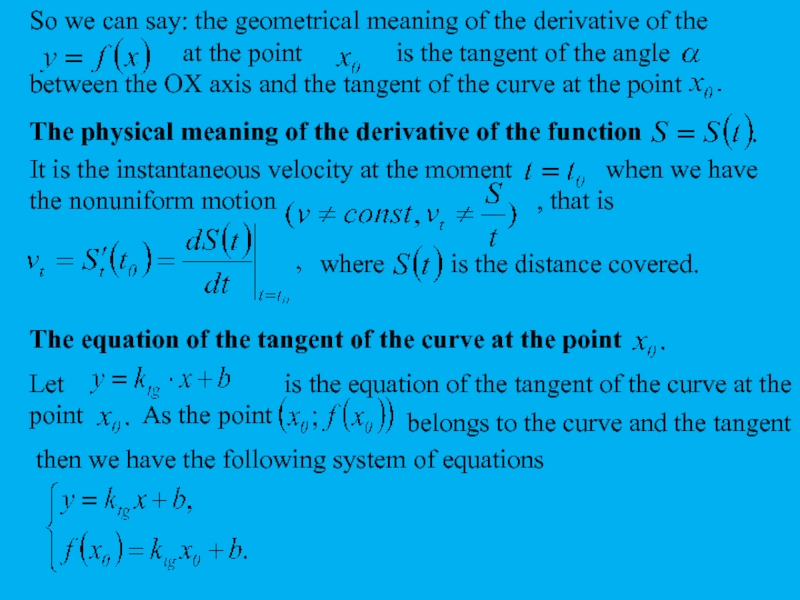
Слайд 4So we can say: the geometrical meaning of the derivative of
at the point is the tangent of the angle
between the OX axis and the tangent of the curve at the point
The physical meaning of the derivative of the function
It is the instantaneous velocity at the moment when we have
the nonuniform motion , that is
The equation of the tangent of the curve at the point
Let is the equation of the tangent of the curve at the
point As the point
belongs to the curve and the tangent
then we have the following system of equations
where is the distance covered.
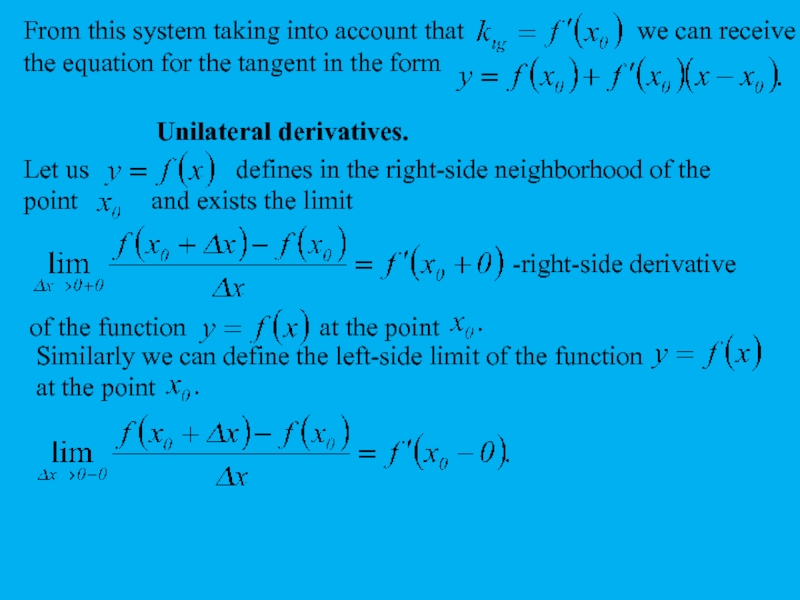
Слайд 5From this system taking into account that
the equation for the tangent in the form
Unilateral derivatives.
Let us defines in the right-side neighborhood of the
point and exists the limit
right-side derivative
of the function at the point
Similarly we can define the left-side limit of the function
at the point
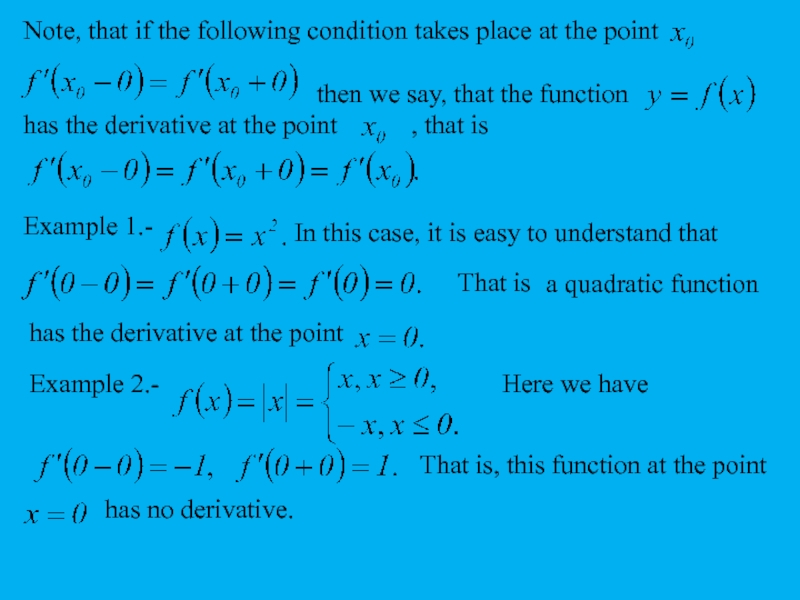
Слайд 6Note, that if the following condition takes place at the point
then we say, that the function
has the derivative at the point , that is
Example 1.-
In this case, it is easy to understand that
That is
a quadratic function
has the derivative at the point
Example 2.-
Here we have
That is, this function at the point
has no derivative.
Слайд 7The difference form of the continuity condition.
Let us consider the
at the point that is or
or
and we have
Differentiability of .
Let defines in the interval and
Слайд 8Definition.-
increment of this function can be presented in a form
where is some
constant, and when
Theorem.- for differentiability of it is necessary and
sufficient, that it had finite derivative.
The proof of necessity.- Let the function is differentiable,
that is or
So we have
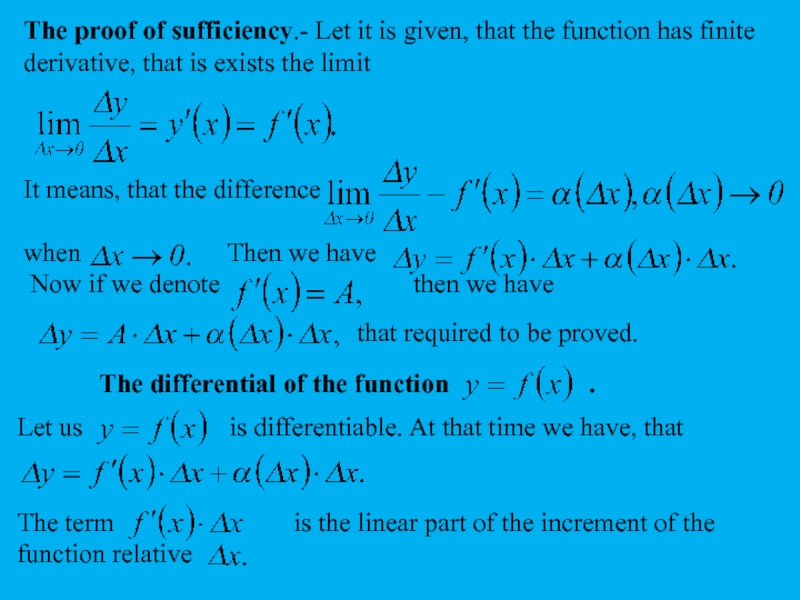
Слайд 9The proof of sufficiency.- Let it is given, that the function
derivative, that is exists the limit
It means, that the difference
when Then we have
Now if we denote then we have
that required to be proved.
The differential of the function .
Let us is differentiable. At that time we have, that
The term is the linear part of the increment of the
function relative
Слайд 10Note, that the quantities
of the same order. But the quantity in compared with
is infinitely small of higher order, that is
So the term is the leading linear part of the increase
of the The term is named the differential
of the function and is indicated as
Note, that if we have the function then we receive
that is So we have
So we can say, that the derivative of the function is equal to the
ratio of and .
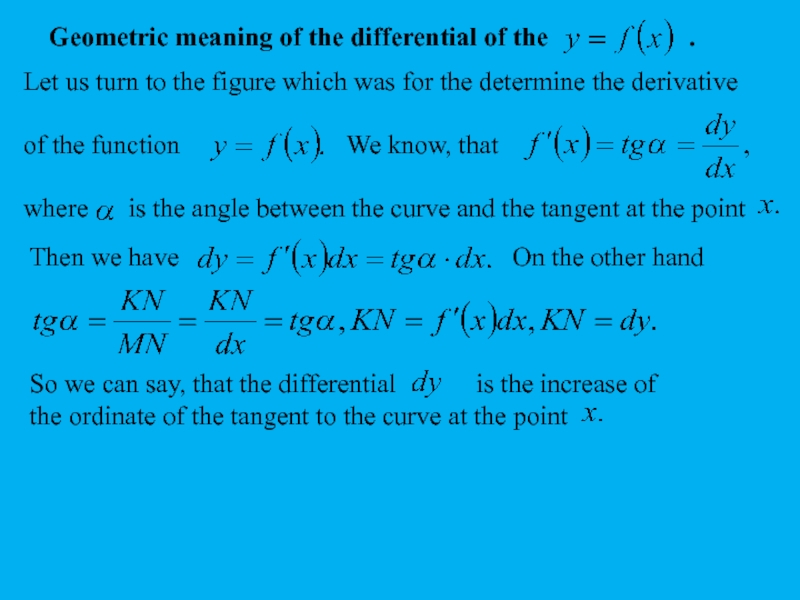
Слайд 11Geometric meaning of the differential of the
Let us turn to the figure which was for the determine the derivative
of the function We know, that
where is the angle between the curve and the tangent at the point
Then we have On the other hand
So we can say, that the differential is the increase of
the ordinate of the tangent to the curve at the point
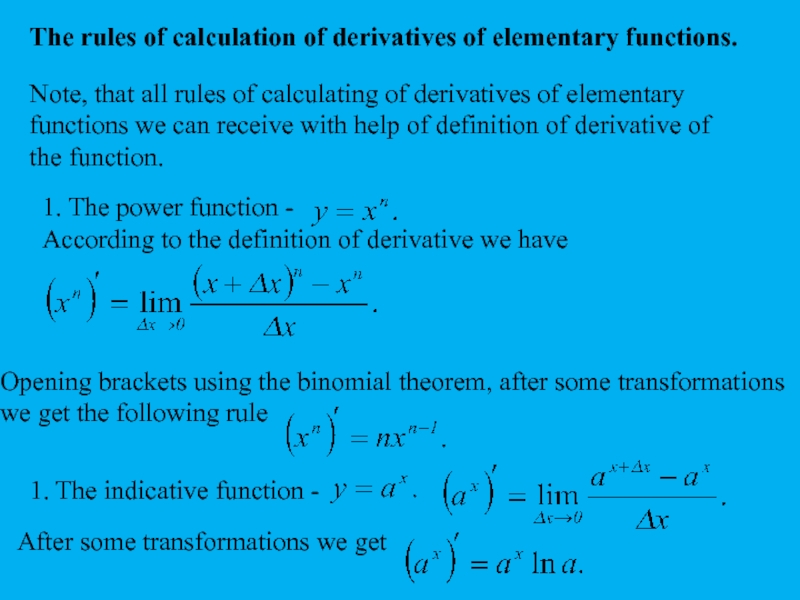
Слайд 12The rules of calculation of derivatives of elementary functions.
Note, that all rules of calculating of derivatives of elementary
functions we can receive with help of definition of derivative of
the function.
1. The power function -
According to the definition of derivative we have
Opening brackets using the binomial theorem, after some transformations
we get the following rule
1. The indicative function -
After some transformations we get