- Главная
- Разное
- Дизайн
- Бизнес и предпринимательство
- Аналитика
- Образование
- Развлечения
- Красота и здоровье
- Финансы
- Государство
- Путешествия
- Спорт
- Недвижимость
- Армия
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Conditional probability and the multiplication rule презентация
Содержание
- 1. Conditional probability and the multiplication rule
- 2. Multiplication Rule The Multiplication Rule can be
- 3. Multiplication Rule Tip Find the probability the
- 4. Using the Multiplication Rule to find Probability
- 5. Using the Multiplication Rule to find Probability
- 6. Using the Multiplication Rule to find Probability
- 7. Using the Multiplication Rule to find Probability
- 8. Using the Multiplication Rule to find Probability
- 9. Using the Multiplication Rule to find Probability
- 10. Using the Multiplication Rule to find Probability
- 11. Using the Multiplication Rule to find Probability
- 12. Dependent Events When the outcome or occurrence
- 13. Conditional Probability To find probabilities when events
- 14. Finding Conditional Probability Two cards
- 15. Finding Conditional Probability Three cards
- 16. Finding Conditional Probability The table at the
- 17. Finding Conditional Probability Find the probability that
Слайд 2Multiplication Rule
The Multiplication Rule can be used to find the probability
of two or more events that occur in a sequence .
The multiplication Rule for the probability of A and B
If events A and B are independent, then the rule can be simplified to P(A and B) = P (A) ● P (B). This simplified rule can be extended for any number of independent events.
The multiplication Rule for the probability of A and B
If events A and B are independent, then the rule can be simplified to P(A and B) = P (A) ● P (B). This simplified rule can be extended for any number of independent events.
Слайд 3Multiplication Rule Tip
Find the probability the first event occurs.
Find the probability
the second event occurs given the first event has occurred and
Multiply these two probabilities.
Multiply these two probabilities.
Слайд 4Using the Multiplication Rule to find Probability
A coin is tossed
and a die is rolled. Find the probability of getting a head and then rolling a 6.
Слайд 5Using the Multiplication Rule to find Probability
A card is drawn
from a deck and replaced; then a second card is drawn. Find the probability of selecting a Ace and then selecting a queen.
Слайд 6Using the Multiplication Rule to find Probability
The probability that a
salmon swims successfully through a dam is 0.85. Find the probability that two salmon successfully swim through the dam.
Слайд 7Using the Multiplication Rule to find Probability
Two cards are selected
from a standard deck without replacement. Find the probability that both are hearts.
Слайд 8Using the Multiplication Rule to find Probability
A Harris poll found
the 46% of Americans say they suffer great stress at least once a week. If three people are selected at random, find the probability that all three will say they suffer great stress at least once a week.
Слайд 9Using the Multiplication Rule to find Probability
The probability that a
salmon swims successfully through a dam is 0.85. Find the probability that three salmon swim successfully through the dam.
Слайд 10Using the Multiplication Rule to find Probability
Find the probability that
none of the three salmon are successful.
Слайд 11Using the Multiplication Rule to find Probability
Find the probability that
at least one of the three salmon is successful in swimming through the dam.
Слайд 12Dependent Events
When the outcome or occurrence of the first event affects
the outcome or occurrence of the second event in such a way that the probability is changed, the events are said to be dependent events.
Examples
Drawing a card from a deck, NOT replacing it, and then drawing a second card.
Being a lifeguard and getting a tan.
Having high grades and getting a scholarship
Examples
Drawing a card from a deck, NOT replacing it, and then drawing a second card.
Being a lifeguard and getting a tan.
Having high grades and getting a scholarship
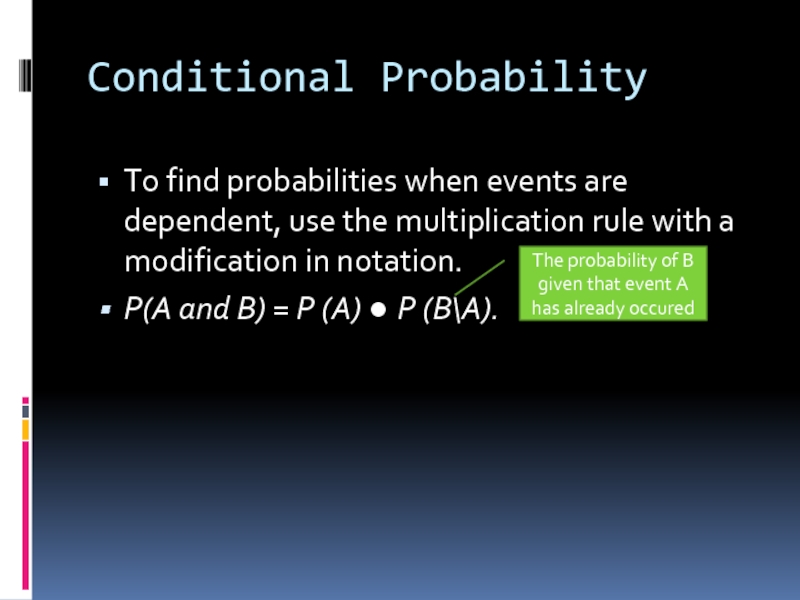
Слайд 13Conditional Probability
To find probabilities when events are dependent, use the multiplication
rule with a modification in notation.
P(A and B) = P (A) ● P (B\A).
P(A and B) = P (A) ● P (B\A).
The probability of B given that event A has already occured
Слайд 14Finding Conditional Probability
Two cards are selected in sequence from
a standard deck. Find the probability that the second card is a queen, given that the first card is a king. (Assume that the king is not replaced)
Solution: Because the first card is a king and is not replaced, the remaining deck has 51 cards, 4 of which are queens. So,
P(B|A) = 4/51 = 0.078
Solution: Because the first card is a king and is not replaced, the remaining deck has 51 cards, 4 of which are queens. So,
P(B|A) = 4/51 = 0.078
Слайд 15Finding Conditional Probability
Three cards are drawn from an ordinary
deck and not replaced. Find the probability of these events.
Getting 3 Jacks
Getting an ace, a king, and a queen in order
Getting a club, a spade, and a heart in order
Getting three clubs
Getting 3 Jacks
Getting an ace, a king, and a queen in order
Getting a club, a spade, and a heart in order
Getting three clubs
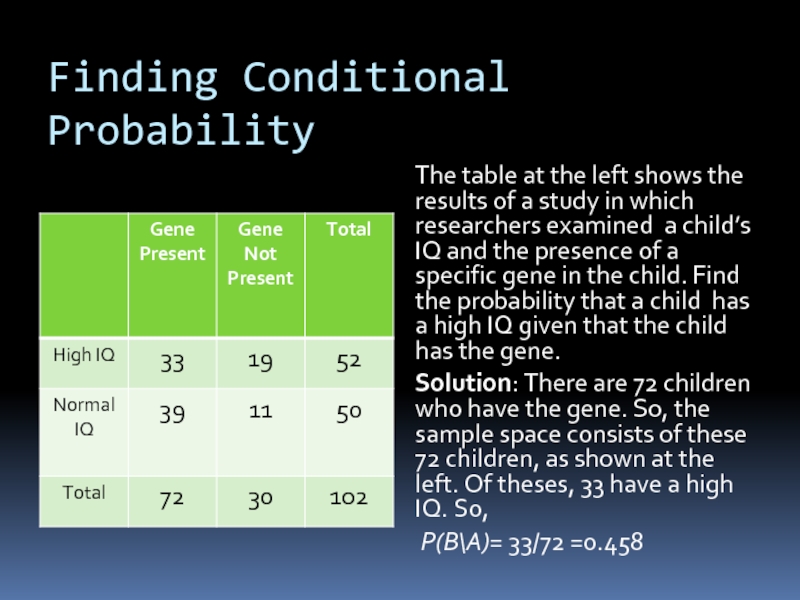
Слайд 16Finding Conditional Probability
The table at the left shows the results of
a study in which researchers examined a child’s IQ and the presence of a specific gene in the child. Find the probability that a child has a high IQ given that the child has the gene.
Solution: There are 72 children who have the gene. So, the sample space consists of these 72 children, as shown at the left. Of theses, 33 have a high IQ. So,
P(B\A)= 33/72 =0.458
Solution: There are 72 children who have the gene. So, the sample space consists of these 72 children, as shown at the left. Of theses, 33 have a high IQ. So,
P(B\A)= 33/72 =0.458
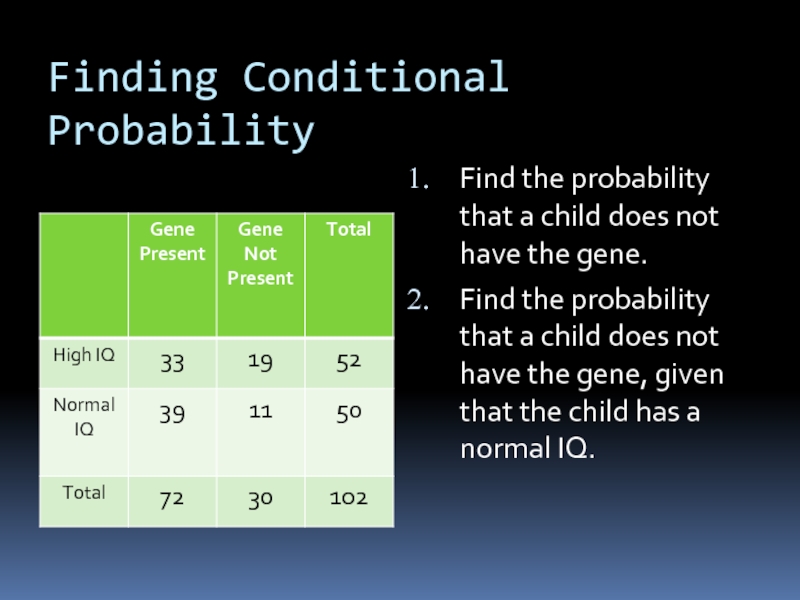
Слайд 17Finding Conditional Probability
Find the probability that a child does not have
the gene.
Find the probability that a child does not have the gene, given that the child has a normal IQ.
Find the probability that a child does not have the gene, given that the child has a normal IQ.