- Главная
- Разное
- Дизайн
- Бизнес и предпринимательство
- Аналитика
- Образование
- Развлечения
- Красота и здоровье
- Финансы
- Государство
- Путешествия
- Спорт
- Недвижимость
- Армия
- Графика
- Культурология
- Еда и кулинария
- Лингвистика
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Детские презентации
- Информатика
- История
- Литература
- Маркетинг
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Equality of two polynomials презентация
Содержание
- 1. Equality of two polynomials
- 2. Equality of two polynomials
- 3. Definition
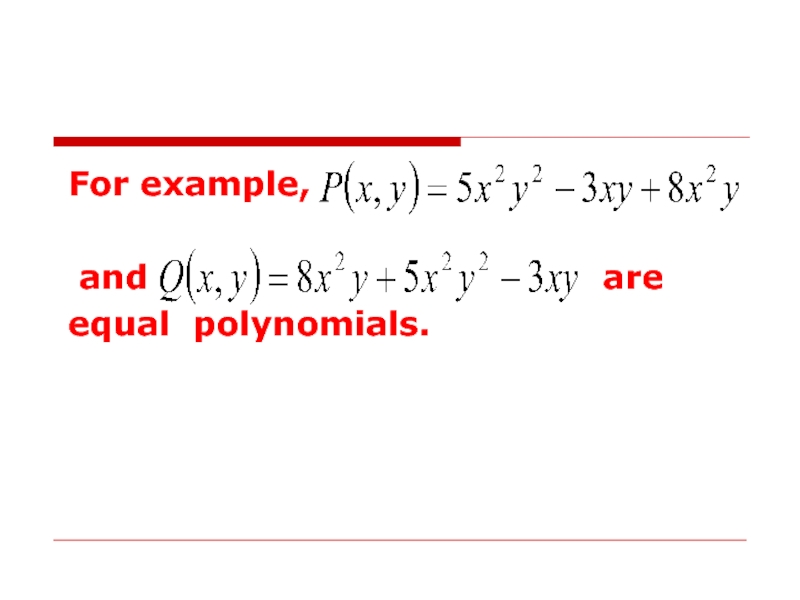
- 4. For example,
- 5. Equal polynomials Two polynomials
- 6. For example,
- 7. 2x2 + 3x = 5
- 8. Recall that the degree of a polynomial
- 9. Let P(x)=anxn+an-1xn-1+…+a2x2+a1x and Q(x)=bmxm+bm-1xm-1+…+b2x2+b1x be
- 10. Example
- 11. Travel to the world. Travel
- 12. Ticket to travel to the world 1 2 3 4
- 26. Pleasant and interesting travel to you Pleasant and interesting travel to you
Слайд 3Definition
1234567891011121314151617181920
If two or more terms contain the same variable (s)
they are called like terms.
Слайд 5
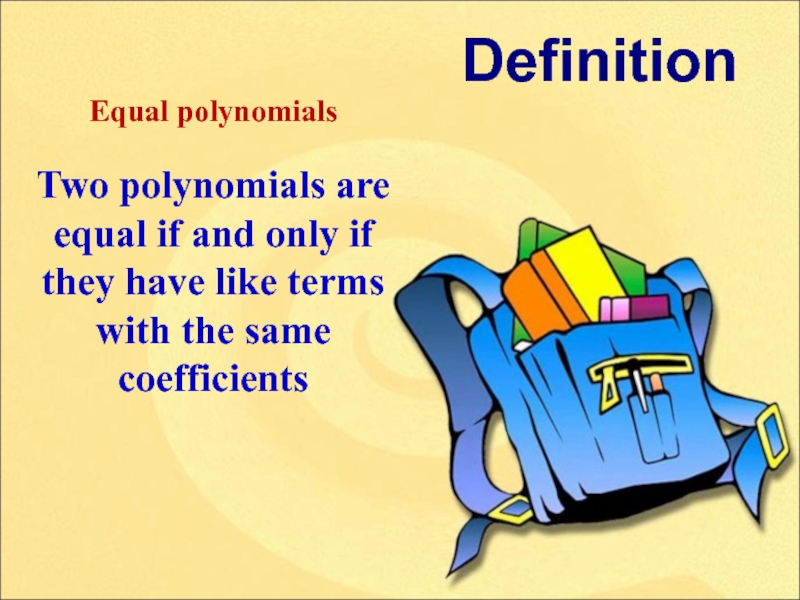
Equal polynomials
Two polynomials are equal if and only if they
have like terms with the same coefficients
Definition
Слайд 7
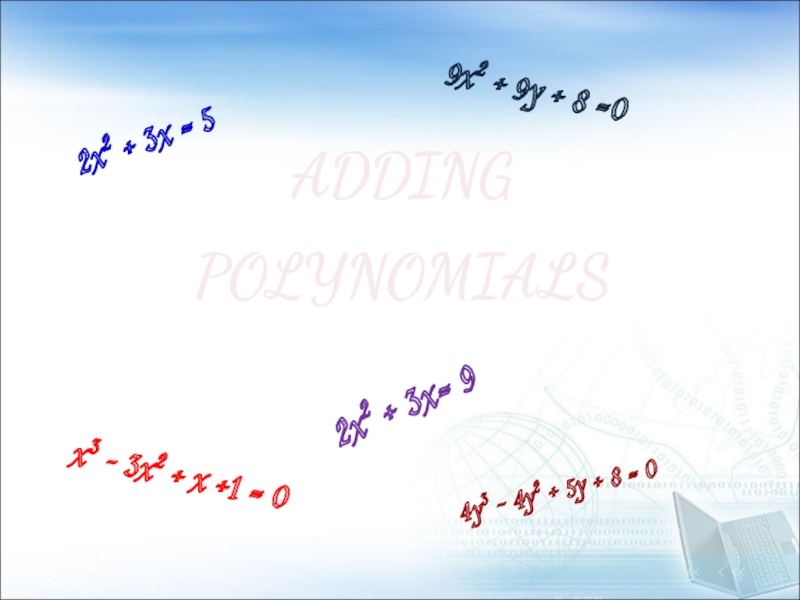
2x2 + 3x = 5
2x2 + 3x= 9
x3 – 3x2
+ x +1 = 0
4y3 - 4y2 + 5y + 8 = 0
9x2 + 9y + 8 =0
ADDING
POLYNOMIALS
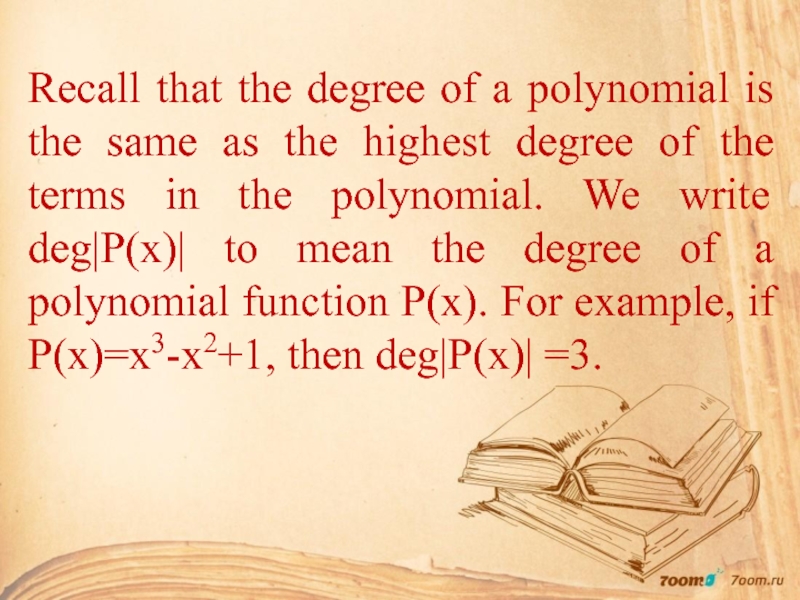
Слайд 8Recall that the degree of a polynomial is the same as
the highest degree of the terms in the polynomial. We write deg|P(x)| to mean the degree of a polynomial function P(x). For example, if P(x)=x3-x2+1, then deg|P(x)| =3.
Слайд 9Let P(x)=anxn+an-1xn-1+…+a2x2+a1x and Q(x)=bmxm+bm-1xm-1+…+b2x2+b1x be two polynomials such that
deg|Q(x)|≥deg|P(x)|. Then the sum of these polynomials is defined as P(x)+Q(x)=bmxm+…+(an+bn)xn+(an-1+bn-1)xn-1+…+(a2+b2)x2+(a1+b1)x+a0+b0
Слайд 10 Example Let P(x)=-6x4+5x3-2x+5, Q(x)=x5+x3+x, and R(x)=2x5+x4-x2 Find each sum. A)
P(x)+Q(x) B) P(x)+R(x) C) R(x)+Q(x)
A) P(x)+Q(x)=x5-6x4+6x3-x+5
B)P(x)+R(x)= 2x5-5x4+5x3- x2 -2x+5
C) R(x)+Q(x)= 3x5-x4+x3- x2 +x